Home / Frost Circles
Aromaticity
Frost Circles
Last updated: October 31st, 2022 |
We’ve spent a lot of time in previous posts “building up” and drawing out the molecular orbitals for various species. In this post we’ll learn an extremely useful shortcut that will help us draw the energy levels of cyclic pi-systems extremely quickly.
The trick is called “Frost Circles”, or, sometimes, the “Polygon method”.
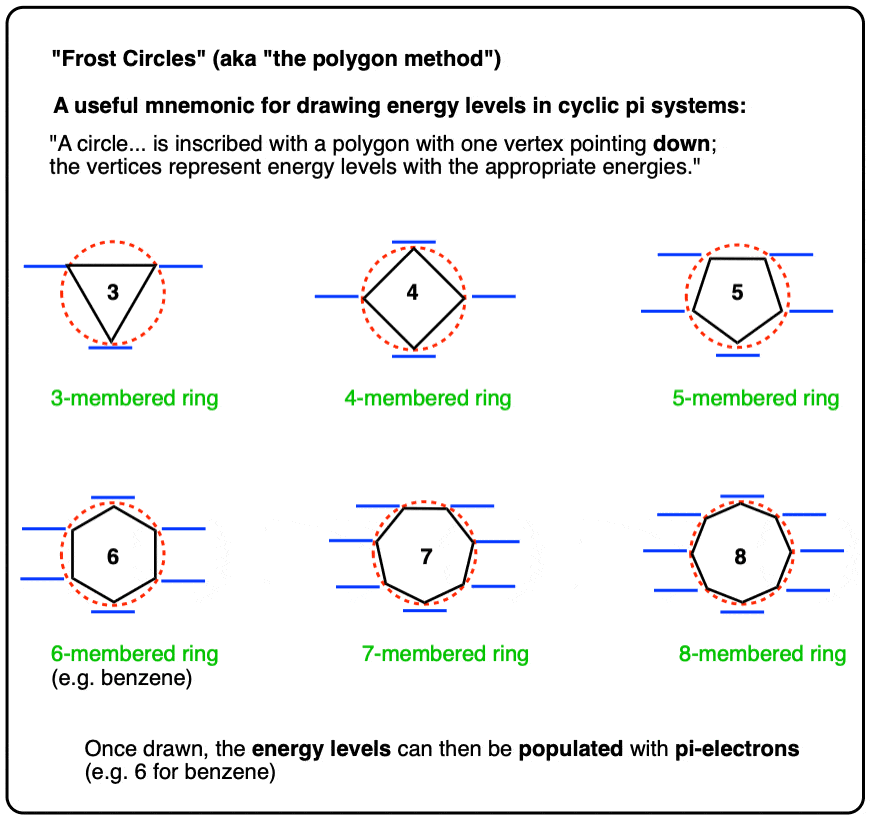
Table of Contents
- The Molecular Orbitals Of Benzene Can Be Superimposed On A Hexagon With the Vertex Pointing Down
- The Molecular Orbitals Of Cyclobutadiene Can Be Superimposed On A Square With the Vertex Pointing Down
- Frost Circles (The Polygon Method): A Useful Mnemonic For Drawing Energy Levels In Cyclic Pi Systems
- Frost Circles For Three-Membered Rings
- Frost Circles For Four-Membered Rings
- Frost Circles For Five-Membered Rings
- Six-Membered Rings
- Seven-Membered Rings
- Eight-Membered Rings
- Summary: Frost Circles
- Notes
- (Advanced) References and Further Reading
1. The Molecular Orbitals Of Benzene Can Be Superimposed On A Hexagon With the Vertex Pointing Down
First: Recall that we saw the energy levels of the molecular orbitals of benzene look like this:
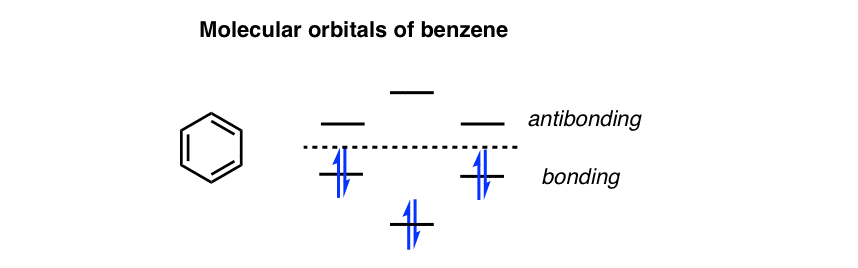
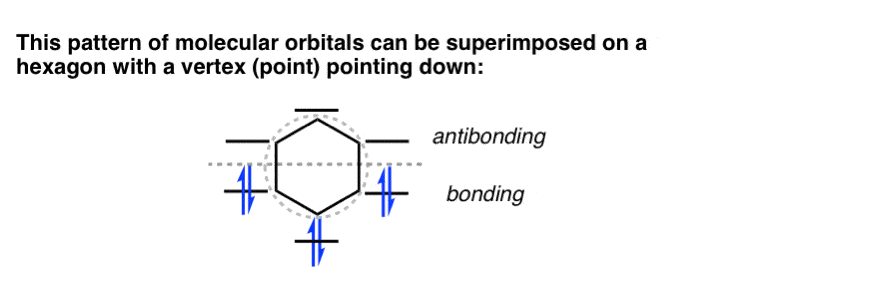
Useful observation: these energy levels can be superimposed on a hexagon with the vertex pointing down. Like this!
Let’s do another one.
2. The Molecular Orbitals Of Cyclobutadiene Can Be Superimposed On A Square With the Vertex Pointing Down
We’ve seen what the molecular orbitals for cyclobutadiene look like; the two highest energy levels are each singly occupied (and non-bonding, to boot) which helps to explain why cyclobutadiene is so spectacularly unstable.
The energy levels for cyclobutadiene can be superimposed on a diamond, which is just a fancy name for a square with its vertex pointing down.
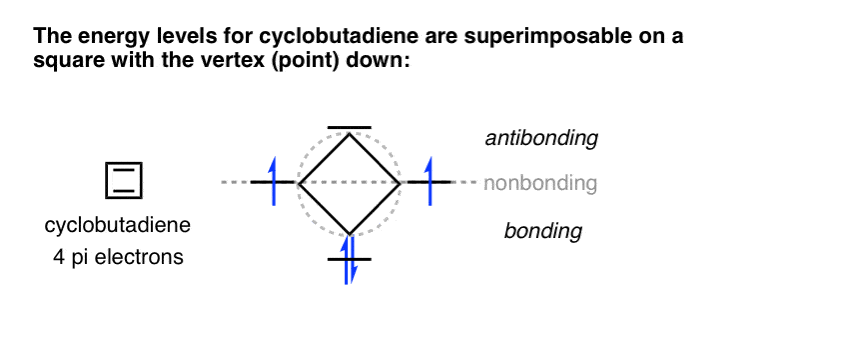
That’s pretty cool. You might wonder…. does it work for other polygons too?
3. Frost Circles (The Polygon Method): A Useful Mnemonic For Drawing Energy Levels In Cyclic Pi Systems
Indeed it does. Back in 1953, Frost published an article describing this method for drawing out the energy levels in cyclic systems, with a simplified version as follows [Note 1]
“A circle… is inscribed with a polygon with one vertex pointing down; the vertices represent energy levels with the appropriate energies”.
Vertices below the halfway mark of the circle are considered bonding orbitals, and vertices above the halfway mark are considered antibonding orbitals. If vertices are exactly in the middle (as they are for 4- and 8- membered rings) they represent non-bonding orbitals.
This idea is presented in the diagram below for 3, 4, 5, 6, 7, and 8-membered rings:
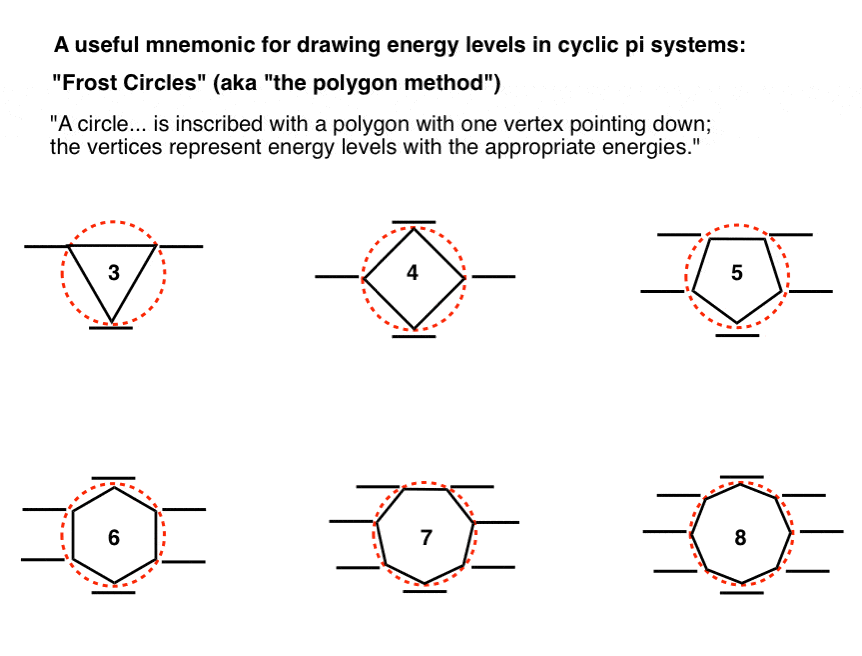
This is an extremely useful mnemonic! This saves us a lot of work in building energy levels from the ground up.
To draw the molecular orbitals of a cyclic pi system, all we have to do is draw the appropriate polygon, vertex-down, and then fill it up with electrons.
Let’s see how we can apply these “Frost Circles” for 3, 4, 5, 6, 7, and 8 membered rings. (Although we’re going to skip drawing the actual circles, and just focus on the positions of the orbitals).
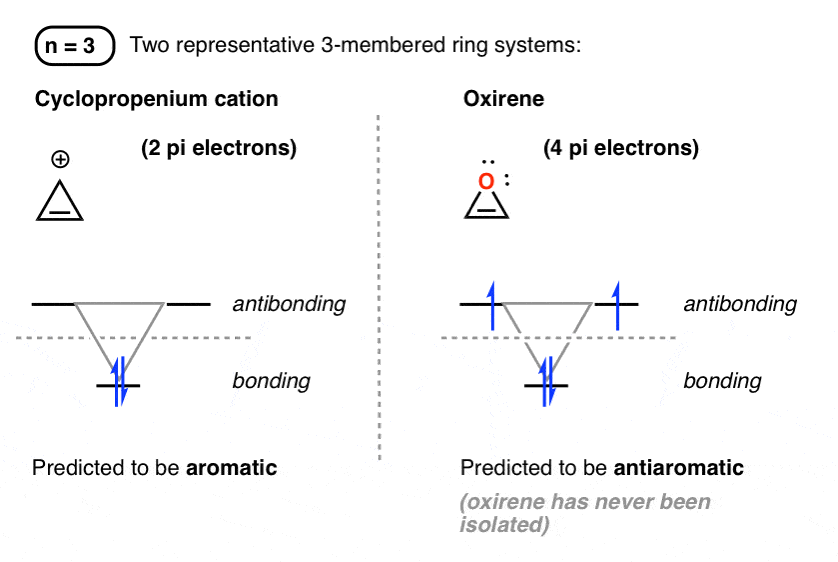
4. Frost Circles For Three-Membered Rings
There are two important configurations of energy levels for 3-membered cyclic pi systems, depending on the number of pi electrons.
With two pi electrons, we’d expect an aromatic molecule. One example is the cyclopropenium cation (below left), which is indeed aromatic.
With 4 pi electrons, an antiaromatic molecule is expected. Oxirene (below right) which has never been isolated, is in this category.
5. Frost Circles For Four-Membered Rings
Cyclobutadiene was covered above, and in the last post. Note that the molecular orbital diagram predicts that if you rip off two of the pi-electrons, the resulting cyclobutene di-cation should be aromatic, (Substituted cyclobutene dications have indeed been synthesized and found to be aromatic, by the group of the estimable, late, George Olah) [Note 2]
6. Frost Circles For Five-Membered Rings
Cyclic 5-membered pi systems with 6 pi electrons are predicted to be aromatic. Examples are abundant. such as the cyclopentadienyl anion (below left), furan (below right), pyrrole, thiophene, imidazole, and many others. And yes, arsoles are aromatic too, but you probably didn’t need me to tell you that.
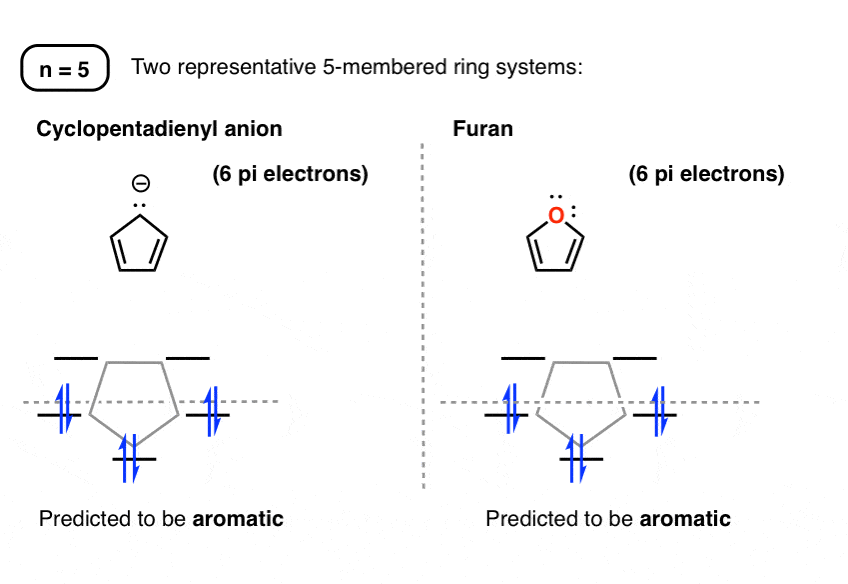
Although not drawn here, removing 2 pi electrons would result in an antiaromatic system. The cyclopentadienyl cation is a classic example.
7. Six-Membered Rings
Already covered above – but note that the rules can be applied not only for benzene, but also “heterocycles” (i.e. aromatic rings with at least one non-carbon ring atom) such as pyridine, pyrimidine, and even the “pyrylium cation”.

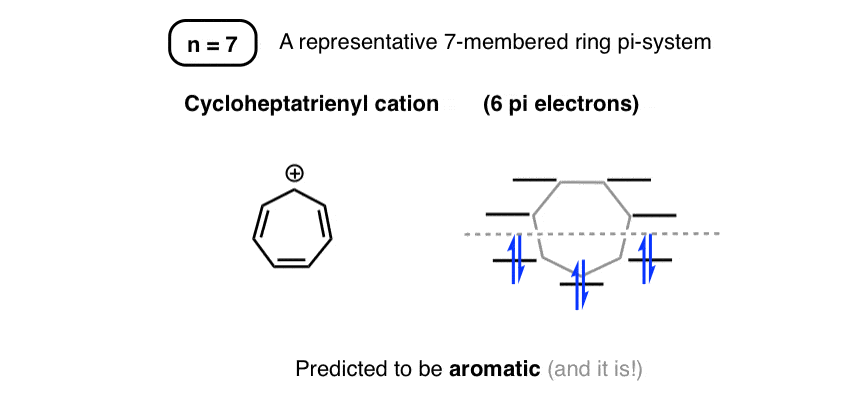
8. Seven-Membered Rings
Cyclic 7-membered pi systems with 6 pi electrons are predicted to be aromatic.
For a ring entirely comprised of carbon atoms, this corresponds to the cycloheptatrienyl cation (sometimes known as the “tropylium ion”).
We’re used to thinking of carbocations as being unstable intermediates with a short lifetime. But the aromatic tropylium ion is such a stable salt you can actually put it in a bottle. Aldrich sells it.
The Frost circle method gives us the energy levels for the tropylium ion:
9. Eight-Membered Rings
With 8 pi electrons, cyclooctatetraene is predicted to be antiaromatic, and its molecular orbitals are predicted to look like this:

If you read the earlier post on antiaromaticity, however, you’ll recall that cyclooctatetraene can “escape” from antiaromaticity by adopting a tub-like shape. The molecular orbital levels we’d predict from the polygon method thus do not exactly correspond to the actual energy levels of cyclooctatetraene itself – it’s not a diradical, for instance.
That’s not quite the end of the story, however. Just like anti aromatic cyclobutadiene can be made aromatic through the removal of two electrons, the stability of (theoretically antiaromatic) cyclooctatetraene can likewise be adjusted by the removal or addition of two pi-electrons to give 6 or 10 pi-electrons, respectively.
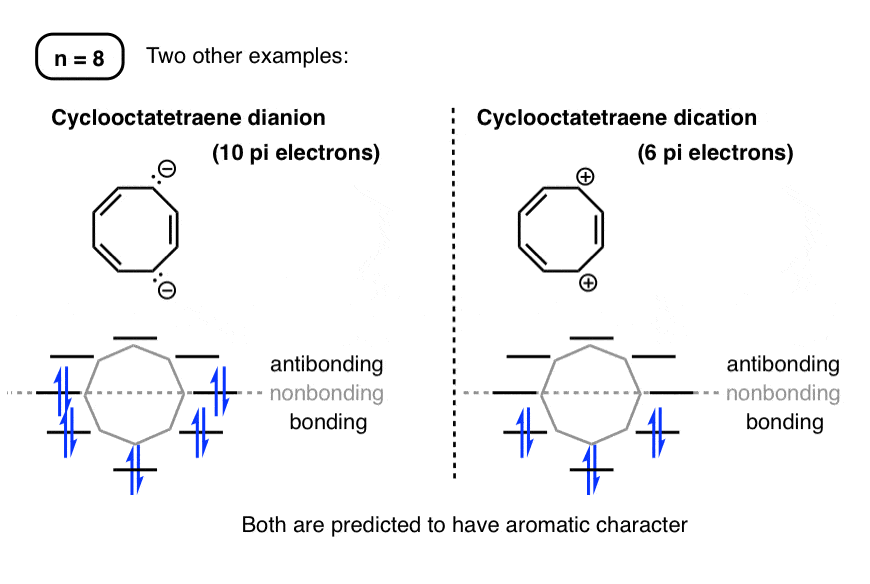
Chemical relatives (“derivatives”) of the cyclooctatetraene dication (6 pi electrons) and the cyclooctatetraene dianion (10 pi electrons) have been synthesized and found to have aromatic character.
Therefore we would predict that these molecules are planar, unlike cyclooctatetraene itself. [In fact, this has been confirmed for the cyclooctatetraene dianion by X-ray crystallography – reference]
10. Summary: Frost Circles
While there’s no end of interesting things to learn about aromaticity (homoaromaticity, anyone?) this post concludes our treatment of it for the time being.
In the next series of articles we’ll finally get in to discussing the reactions of aromatic compounds, beginning with the most important reaction type of all: electrophilic aromatic substitution.
Many thanks to Matt Knowe for assistance with this article.
Notes
Related Articles
- Aromatic, Non-Aromatic, or Antiaromatic? Some Practice Problems
- The Pi Molecular Orbitals of Benzene
- The Pi Molecular Orbitals of Cyclobutadiene
- Molecular Orbitals of The Allyl Cation, Allyl Radical, and Allyl Anion
- Aromaticity Practice Quizzes (MOC Membership)
- Introduction To Aromaticity
- Electrophilic Aromatic Substitutions (1) – Halogenation of Benzene
- Electrophilic Aromatic Substitution – The Mechanism
Note 1. The more complex version is:
A circle centered at α with radius 2β is inscribed with a polygon with one vertex pointing down; the vertices represent energy levels with the appropriate energies
What are these terms “α” and “2β”, you may ask? The answer is beyond the scope of what we’ll discuss here, but the essence is that they are important terms in Hückel Molecular Orbital Method, a way of simplifying the Schrödinger Wave Equation for pi systems by treating the pi electrons and sigma electrons separately. It’s a powerful and useful method for calculating the energies of pi systems and understanding their reactions.
Note 2. The stability arising from aromaticity in the cyclobutadiene dication should be reduced considerably, however, by the Coulombic repulsion arising from the di-cationic nature of the molecule (like charges repel, after all). Similarly, the stability arising from aromaticity of the cyclooctatetraene dianion is lessened somewhat by the repulsion of its two negative charges.
(Advanced) References and Further Reading
Synthesis and Isolation of cyclooctatetraene dianion:
- THE CYCLOÖCTATETRAENYL DIANION
Thomas J. KatzJournal of the American Chemical Society 1960 82 (14), 3784-3785
DOI: 10.1021/ja01499a077
In this paper Prof. Thomas Katz of Columbia University shows how the cyclooctatetraene dianion can be prepared by reducing cyclooctatetraene with two equivalents of potassium metal. -
Structure of the 10.pi. electron cyclooctatetraene dianion in potassium diglyme 1,3,5,7-tetramethylcyclooctatetraene dianion, [K((CH3OCH2CH2)2O)]2[C8H4(CH3)4]
Stephen Z. Goldberg, Kenneth N. Raymond, C. A. Harmon, and David H. TempletonJournal of the American Chemical Society 1974 96 (5), 1348-1351
DOI: 10.1021/ja00812a015
This 1974 work has the first published crystal structure of the cyclooctatetraene dianion and show that it is indeed planar and has structural features consistent with aromaticity. - Free Cyclooctatetraene Dianion: Planarity, Aromaticity, and Theoretical Challenges
Alexander Yu. Sokolov, D. Brandon Magers, Judy I. Wu, Wesley D. Allen, Paul v. R. Schleyer, and Henry F. Schaefer, IIIJournal of Chemical Theory and Computation 2013 9 (10), 4436-4443
DOI: 10.1021/ct400642y
So is cyclooctatetraene actually aromatic? This paper suggests that while the cyclooctatetraene dianion has considerable resonance stabilization (25 kcal/mol, vs. about 33 kcal/mol for benzene) this stabilization is swamped by Coulombic repulsion of the anions, and as noted by commenter Frosty the Circle, : “Like many multiply charged anions, COT2− exists in isolation only… as a short-lived resonance state lying above neutral COT” “….Charge-compensating complexation of COT2−with two sodium cations results in a thermodynamically stable Na2COT compound”
00 General Chemistry Review
01 Bonding, Structure, and Resonance
- How Do We Know Methane (CH4) Is Tetrahedral?
- Hybrid Orbitals and Hybridization
- How To Determine Hybridization: A Shortcut
- Orbital Hybridization And Bond Strengths
- Sigma bonds come in six varieties: Pi bonds come in one
- A Key Skill: How to Calculate Formal Charge
- The Four Intermolecular Forces and How They Affect Boiling Points
- 3 Trends That Affect Boiling Points
- How To Use Electronegativity To Determine Electron Density (and why NOT to trust formal charge)
- Introduction to Resonance
- How To Use Curved Arrows To Interchange Resonance Forms
- Evaluating Resonance Forms (1) - The Rule of Least Charges
- How To Find The Best Resonance Structure By Applying Electronegativity
- Evaluating Resonance Structures With Negative Charges
- Evaluating Resonance Structures With Positive Charge
- Exploring Resonance: Pi-Donation
- Exploring Resonance: Pi-acceptors
- In Summary: Evaluating Resonance Structures
- Drawing Resonance Structures: 3 Common Mistakes To Avoid
- How to apply electronegativity and resonance to understand reactivity
- Bond Hybridization Practice
- Structure and Bonding Practice Quizzes
- Resonance Structures Practice
02 Acid Base Reactions
- Introduction to Acid-Base Reactions
- Acid Base Reactions In Organic Chemistry
- The Stronger The Acid, The Weaker The Conjugate Base
- Walkthrough of Acid-Base Reactions (3) - Acidity Trends
- Five Key Factors That Influence Acidity
- Acid-Base Reactions: Introducing Ka and pKa
- How to Use a pKa Table
- The pKa Table Is Your Friend
- A Handy Rule of Thumb for Acid-Base Reactions
- Acid Base Reactions Are Fast
- pKa Values Span 60 Orders Of Magnitude
- How Protonation and Deprotonation Affect Reactivity
- Acid Base Practice Problems
03 Alkanes and Nomenclature
- Meet the (Most Important) Functional Groups
- Condensed Formulas: Deciphering What the Brackets Mean
- Hidden Hydrogens, Hidden Lone Pairs, Hidden Counterions
- Don't Be Futyl, Learn The Butyls
- Primary, Secondary, Tertiary, Quaternary In Organic Chemistry
- Branching, and Its Affect On Melting and Boiling Points
- The Many, Many Ways of Drawing Butane
- Wedge And Dash Convention For Tetrahedral Carbon
- Common Mistakes in Organic Chemistry: Pentavalent Carbon
- Table of Functional Group Priorities for Nomenclature
- Summary Sheet - Alkane Nomenclature
- Organic Chemistry IUPAC Nomenclature Demystified With A Simple Puzzle Piece Approach
- Boiling Point Quizzes
- Organic Chemistry Nomenclature Quizzes
04 Conformations and Cycloalkanes
- Staggered vs Eclipsed Conformations of Ethane
- Conformational Isomers of Propane
- Newman Projection of Butane (and Gauche Conformation)
- Introduction to Cycloalkanes (1)
- Geometric Isomers In Small Rings: Cis And Trans Cycloalkanes
- Calculation of Ring Strain In Cycloalkanes
- Cycloalkanes - Ring Strain In Cyclopropane And Cyclobutane
- Cyclohexane Conformations
- Cyclohexane Chair Conformation: An Aerial Tour
- How To Draw The Cyclohexane Chair Conformation
- The Cyclohexane Chair Flip
- The Cyclohexane Chair Flip - Energy Diagram
- Substituted Cyclohexanes - Axial vs Equatorial
- Ranking The Bulkiness Of Substituents On Cyclohexanes: "A-Values"
- Cyclohexane Chair Conformation Stability: Which One Is Lower Energy?
- Fused Rings - Cis-Decalin and Trans-Decalin
- Naming Bicyclic Compounds - Fused, Bridged, and Spiro
- Bredt's Rule (And Summary of Cycloalkanes)
- Newman Projection Practice
- Cycloalkanes Practice Problems
05 A Primer On Organic Reactions
- The Most Important Question To Ask When Learning a New Reaction
- Learning New Reactions: How Do The Electrons Move?
- The Third Most Important Question to Ask When Learning A New Reaction
- 7 Factors that stabilize negative charge in organic chemistry
- 7 Factors That Stabilize Positive Charge in Organic Chemistry
- Nucleophiles and Electrophiles
- Curved Arrows (for reactions)
- Curved Arrows (2): Initial Tails and Final Heads
- Nucleophilicity vs. Basicity
- The Three Classes of Nucleophiles
- What Makes A Good Nucleophile?
- What makes a good leaving group?
- 3 Factors That Stabilize Carbocations
- Equilibrium and Energy Relationships
- What's a Transition State?
- Hammond's Postulate
- Learning Organic Chemistry Reactions: A Checklist (PDF)
- Introduction to Free Radical Substitution Reactions
- Introduction to Oxidative Cleavage Reactions
06 Free Radical Reactions
- Bond Dissociation Energies = Homolytic Cleavage
- Free Radical Reactions
- 3 Factors That Stabilize Free Radicals
- What Factors Destabilize Free Radicals?
- Bond Strengths And Radical Stability
- Free Radical Initiation: Why Is "Light" Or "Heat" Required?
- Initiation, Propagation, Termination
- Monochlorination Products Of Propane, Pentane, And Other Alkanes
- Selectivity In Free Radical Reactions
- Selectivity in Free Radical Reactions: Bromination vs. Chlorination
- Halogenation At Tiffany's
- Allylic Bromination
- Bonus Topic: Allylic Rearrangements
- In Summary: Free Radicals
- Synthesis (2) - Reactions of Alkanes
- Free Radicals Practice Quizzes
07 Stereochemistry and Chirality
- Types of Isomers: Constitutional Isomers, Stereoisomers, Enantiomers, and Diastereomers
- How To Draw The Enantiomer Of A Chiral Molecule
- How To Draw A Bond Rotation
- Introduction to Assigning (R) and (S): The Cahn-Ingold-Prelog Rules
- Assigning Cahn-Ingold-Prelog (CIP) Priorities (2) - The Method of Dots
- Enantiomers vs Diastereomers vs The Same? Two Methods For Solving Problems
- Assigning R/S To Newman Projections (And Converting Newman To Line Diagrams)
- How To Determine R and S Configurations On A Fischer Projection
- The Meso Trap
- Optical Rotation, Optical Activity, and Specific Rotation
- Optical Purity and Enantiomeric Excess
- What's a Racemic Mixture?
- Chiral Allenes And Chiral Axes
- Stereochemistry Practice Problems and Quizzes
08 Substitution Reactions
- Introduction to Nucleophilic Substitution Reactions
- Walkthrough of Substitution Reactions (1) - Introduction
- Two Types of Nucleophilic Substitution Reactions
- The SN2 Mechanism
- Why the SN2 Reaction Is Powerful
- The SN1 Mechanism
- The Conjugate Acid Is A Better Leaving Group
- Comparing the SN1 and SN2 Reactions
- Polar Protic? Polar Aprotic? Nonpolar? All About Solvents
- Steric Hindrance is Like a Fat Goalie
- Common Blind Spot: Intramolecular Reactions
- The Conjugate Base is Always a Stronger Nucleophile
- Substitution Practice - SN1
- Substitution Practice - SN2
09 Elimination Reactions
- Elimination Reactions (1): Introduction And The Key Pattern
- Elimination Reactions (2): The Zaitsev Rule
- Elimination Reactions Are Favored By Heat
- Two Elimination Reaction Patterns
- The E1 Reaction
- The E2 Mechanism
- E1 vs E2: Comparing the E1 and E2 Reactions
- Antiperiplanar Relationships: The E2 Reaction and Cyclohexane Rings
- Bulky Bases in Elimination Reactions
- Comparing the E1 vs SN1 Reactions
- Elimination (E1) Reactions With Rearrangements
- E1cB - Elimination (Unimolecular) Conjugate Base
- Elimination (E1) Practice Problems And Solutions
- Elimination (E2) Practice Problems and Solutions
10 Rearrangements
11 SN1/SN2/E1/E2 Decision
- Identifying Where Substitution and Elimination Reactions Happen
- Deciding SN1/SN2/E1/E2 (1) - The Substrate
- Deciding SN1/SN2/E1/E2 (2) - The Nucleophile/Base
- SN1 vs E1 and SN2 vs E2 : The Temperature
- Deciding SN1/SN2/E1/E2 - The Solvent
- Wrapup: The Key Factors For Determining SN1/SN2/E1/E2
- Alkyl Halide Reaction Map And Summary
- SN1 SN2 E1 E2 Practice Problems
12 Alkene Reactions
- E and Z Notation For Alkenes (+ Cis/Trans)
- Alkene Stability
- Alkene Addition Reactions: "Regioselectivity" and "Stereoselectivity" (Syn/Anti)
- Stereoselective and Stereospecific Reactions
- Hydrohalogenation of Alkenes and Markovnikov's Rule
- Hydration of Alkenes With Aqueous Acid
- Rearrangements in Alkene Addition Reactions
- Halogenation of Alkenes and Halohydrin Formation
- Oxymercuration Demercuration of Alkenes
- Hydroboration Oxidation of Alkenes
- m-CPBA (meta-chloroperoxybenzoic acid)
- OsO4 (Osmium Tetroxide) for Dihydroxylation of Alkenes
- Palladium on Carbon (Pd/C) for Catalytic Hydrogenation of Alkenes
- Cyclopropanation of Alkenes
- A Fourth Alkene Addition Pattern - Free Radical Addition
- Alkene Reactions: Ozonolysis
- Summary: Three Key Families Of Alkene Reaction Mechanisms
- Synthesis (4) - Alkene Reaction Map, Including Alkyl Halide Reactions
- Alkene Reactions Practice Problems
13 Alkyne Reactions
- Acetylides from Alkynes, And Substitution Reactions of Acetylides
- Partial Reduction of Alkynes With Lindlar's Catalyst
- Partial Reduction of Alkynes With Na/NH3 To Obtain Trans Alkenes
- Alkyne Hydroboration With "R2BH"
- Hydration and Oxymercuration of Alkynes
- Hydrohalogenation of Alkynes
- Alkyne Halogenation: Bromination, Chlorination, and Iodination of Alkynes
- Alkyne Reactions - The "Concerted" Pathway
- Alkenes To Alkynes Via Halogenation And Elimination Reactions
- Alkynes Are A Blank Canvas
- Synthesis (5) - Reactions of Alkynes
- Alkyne Reactions Practice Problems With Answers
14 Alcohols, Epoxides and Ethers
- Alcohols - Nomenclature and Properties
- Alcohols Can Act As Acids Or Bases (And Why It Matters)
- Alcohols - Acidity and Basicity
- The Williamson Ether Synthesis
- Ethers From Alkenes, Tertiary Alkyl Halides and Alkoxymercuration
- Alcohols To Ethers via Acid Catalysis
- Cleavage Of Ethers With Acid
- Epoxides - The Outlier Of The Ether Family
- Opening of Epoxides With Acid
- Epoxide Ring Opening With Base
- Making Alkyl Halides From Alcohols
- Tosylates And Mesylates
- PBr3 and SOCl2
- Elimination Reactions of Alcohols
- Elimination of Alcohols To Alkenes With POCl3
- Alcohol Oxidation: "Strong" and "Weak" Oxidants
- Demystifying The Mechanisms of Alcohol Oxidations
- Protecting Groups For Alcohols
- Thiols And Thioethers
- Calculating the oxidation state of a carbon
- Oxidation and Reduction in Organic Chemistry
- Oxidation Ladders
- SOCl2 Mechanism For Alcohols To Alkyl Halides: SN2 versus SNi
- Alcohol Reactions Roadmap (PDF)
- Alcohol Reaction Practice Problems
- Epoxide Reaction Quizzes
- Oxidation and Reduction Practice Quizzes
15 Organometallics
- What's An Organometallic?
- Formation of Grignard and Organolithium Reagents
- Organometallics Are Strong Bases
- Reactions of Grignard Reagents
- Protecting Groups In Grignard Reactions
- Synthesis Problems Involving Grignard Reagents
- Grignard Reactions And Synthesis (2)
- Organocuprates (Gilman Reagents): How They're Made
- Gilman Reagents (Organocuprates): What They're Used For
- The Heck, Suzuki, and Olefin Metathesis Reactions (And Why They Don't Belong In Most Introductory Organic Chemistry Courses)
- Reaction Map: Reactions of Organometallics
- Grignard Practice Problems
16 Spectroscopy
- Degrees of Unsaturation (or IHD, Index of Hydrogen Deficiency)
- Conjugation And Color (+ How Bleach Works)
- Introduction To UV-Vis Spectroscopy
- UV-Vis Spectroscopy: Absorbance of Carbonyls
- UV-Vis Spectroscopy: Practice Questions
- Bond Vibrations, Infrared Spectroscopy, and the "Ball and Spring" Model
- Infrared Spectroscopy: A Quick Primer On Interpreting Spectra
- IR Spectroscopy: 4 Practice Problems
- 1H NMR: How Many Signals?
- Homotopic, Enantiotopic, Diastereotopic
- Diastereotopic Protons in 1H NMR Spectroscopy: Examples
- C13 NMR - How Many Signals
- Liquid Gold: Pheromones In Doe Urine
- Natural Product Isolation (1) - Extraction
- Natural Product Isolation (2) - Purification Techniques, An Overview
- Structure Determination Case Study: Deer Tarsal Gland Pheromone
17 Dienes and MO Theory
- What To Expect In Organic Chemistry 2
- Are these molecules conjugated?
- Conjugation And Resonance In Organic Chemistry
- Bonding And Antibonding Pi Orbitals
- Molecular Orbitals of The Allyl Cation, Allyl Radical, and Allyl Anion
- Pi Molecular Orbitals of Butadiene
- Reactions of Dienes: 1,2 and 1,4 Addition
- Thermodynamic and Kinetic Products
- More On 1,2 and 1,4 Additions To Dienes
- s-cis and s-trans
- The Diels-Alder Reaction
- Cyclic Dienes and Dienophiles in the Diels-Alder Reaction
- Stereochemistry of the Diels-Alder Reaction
- Exo vs Endo Products In The Diels Alder: How To Tell Them Apart
- HOMO and LUMO In the Diels Alder Reaction
- Why Are Endo vs Exo Products Favored in the Diels-Alder Reaction?
- Diels-Alder Reaction: Kinetic and Thermodynamic Control
- The Retro Diels-Alder Reaction
- The Intramolecular Diels Alder Reaction
- Regiochemistry In The Diels-Alder Reaction
- The Cope and Claisen Rearrangements
- Electrocyclic Reactions
- Electrocyclic Ring Opening And Closure (2) - Six (or Eight) Pi Electrons
- Diels Alder Practice Problems
- Molecular Orbital Theory Practice
18 Aromaticity
- Introduction To Aromaticity
- Rules For Aromaticity
- Huckel's Rule: What Does 4n+2 Mean?
- Aromatic, Non-Aromatic, or Antiaromatic? Some Practice Problems
- Antiaromatic Compounds and Antiaromaticity
- The Pi Molecular Orbitals of Benzene
- The Pi Molecular Orbitals of Cyclobutadiene
- Frost Circles
- Aromaticity Practice Quizzes
19 Reactions of Aromatic Molecules
- Electrophilic Aromatic Substitution: Introduction
- Activating and Deactivating Groups In Electrophilic Aromatic Substitution
- Electrophilic Aromatic Substitution - The Mechanism
- Ortho-, Para- and Meta- Directors in Electrophilic Aromatic Substitution
- Understanding Ortho, Para, and Meta Directors
- Why are halogens ortho- para- directors?
- Disubstituted Benzenes: The Strongest Electron-Donor "Wins"
- Electrophilic Aromatic Substitutions (1) - Halogenation of Benzene
- Electrophilic Aromatic Substitutions (2) - Nitration and Sulfonation
- EAS Reactions (3) - Friedel-Crafts Acylation and Friedel-Crafts Alkylation
- Intramolecular Friedel-Crafts Reactions
- Nucleophilic Aromatic Substitution (NAS)
- Nucleophilic Aromatic Substitution (2) - The Benzyne Mechanism
- Reactions on the "Benzylic" Carbon: Bromination And Oxidation
- The Wolff-Kishner, Clemmensen, And Other Carbonyl Reductions
- More Reactions on the Aromatic Sidechain: Reduction of Nitro Groups and the Baeyer Villiger
- Aromatic Synthesis (1) - "Order Of Operations"
- Synthesis of Benzene Derivatives (2) - Polarity Reversal
- Aromatic Synthesis (3) - Sulfonyl Blocking Groups
- Birch Reduction
- Synthesis (7): Reaction Map of Benzene and Related Aromatic Compounds
- Aromatic Reactions and Synthesis Practice
- Electrophilic Aromatic Substitution Practice Problems
20 Aldehydes and Ketones
- What's The Alpha Carbon In Carbonyl Compounds?
- Nucleophilic Addition To Carbonyls
- Aldehydes and Ketones: 14 Reactions With The Same Mechanism
- Sodium Borohydride (NaBH4) Reduction of Aldehydes and Ketones
- Grignard Reagents For Addition To Aldehydes and Ketones
- Wittig Reaction
- Hydrates, Hemiacetals, and Acetals
- Imines - Properties, Formation, Reactions, and Mechanisms
- All About Enamines
- Breaking Down Carbonyl Reaction Mechanisms: Reactions of Anionic Nucleophiles (Part 2)
- Aldehydes Ketones Reaction Practice
21 Carboxylic Acid Derivatives
- Nucleophilic Acyl Substitution (With Negatively Charged Nucleophiles)
- Addition-Elimination Mechanisms With Neutral Nucleophiles (Including Acid Catalysis)
- Basic Hydrolysis of Esters - Saponification
- Transesterification
- Proton Transfer
- Fischer Esterification - Carboxylic Acid to Ester Under Acidic Conditions
- Lithium Aluminum Hydride (LiAlH4) For Reduction of Carboxylic Acid Derivatives
- LiAlH[Ot-Bu]3 For The Reduction of Acid Halides To Aldehydes
- Di-isobutyl Aluminum Hydride (DIBAL) For The Partial Reduction of Esters and Nitriles
- Amide Hydrolysis
- Thionyl Chloride (SOCl2)
- Diazomethane (CH2N2)
- Carbonyl Chemistry: Learn Six Mechanisms For the Price Of One
- Making Music With Mechanisms (PADPED)
- Carboxylic Acid Derivatives Practice Questions
22 Enols and Enolates
- Keto-Enol Tautomerism
- Enolates - Formation, Stability, and Simple Reactions
- Kinetic Versus Thermodynamic Enolates
- Aldol Addition and Condensation Reactions
- Reactions of Enols - Acid-Catalyzed Aldol, Halogenation, and Mannich Reactions
- Claisen Condensation and Dieckmann Condensation
- Decarboxylation
- The Malonic Ester and Acetoacetic Ester Synthesis
- The Michael Addition Reaction and Conjugate Addition
- The Robinson Annulation
- Haloform Reaction
- The Hell–Volhard–Zelinsky Reaction
- Enols and Enolates Practice Quizzes
23 Amines
- The Amide Functional Group: Properties, Synthesis, and Nomenclature
- Basicity of Amines And pKaH
- 5 Key Basicity Trends of Amines
- The Mesomeric Effect And Aromatic Amines
- Nucleophilicity of Amines
- Alkylation of Amines (Sucks!)
- Reductive Amination
- The Gabriel Synthesis
- Some Reactions of Azides
- The Hofmann Elimination
- The Hofmann and Curtius Rearrangements
- The Cope Elimination
- Protecting Groups for Amines - Carbamates
- The Strecker Synthesis of Amino Acids
- Introduction to Peptide Synthesis
- Reactions of Diazonium Salts: Sandmeyer and Related Reactions
- Amine Practice Questions
24 Carbohydrates
- D and L Notation For Sugars
- Pyranoses and Furanoses: Ring-Chain Tautomerism In Sugars
- What is Mutarotation?
- Reducing Sugars
- The Big Damn Post Of Carbohydrate-Related Chemistry Definitions
- The Haworth Projection
- Converting a Fischer Projection To A Haworth (And Vice Versa)
- Reactions of Sugars: Glycosylation and Protection
- The Ruff Degradation and Kiliani-Fischer Synthesis
- Isoelectric Points of Amino Acids (and How To Calculate Them)
- Carbohydrates Practice
- Amino Acid Quizzes
25 Fun and Miscellaneous
- A Gallery of Some Interesting Molecules From Nature
- Screw Organic Chemistry, I'm Just Going To Write About Cats
- On Cats, Part 1: Conformations and Configurations
- On Cats, Part 2: Cat Line Diagrams
- On Cats, Part 4: Enantiocats
- On Cats, Part 6: Stereocenters
- Organic Chemistry Is Shit
- The Organic Chemistry Behind "The Pill"
- Maybe they should call them, "Formal Wins" ?
- Why Do Organic Chemists Use Kilocalories?
- The Principle of Least Effort
- Organic Chemistry GIFS - Resonance Forms
- Reproducibility In Organic Chemistry
- What Holds The Nucleus Together?
- How Reactions Are Like Music
- Organic Chemistry and the New MCAT
26 Organic Chemistry Tips and Tricks
- Common Mistakes: Formal Charges Can Mislead
- Partial Charges Give Clues About Electron Flow
- Draw The Ugly Version First
- Organic Chemistry Study Tips: Learn the Trends
- The 8 Types of Arrows In Organic Chemistry, Explained
- Top 10 Skills To Master Before An Organic Chemistry 2 Final
- Common Mistakes with Carbonyls: Carboxylic Acids... Are Acids!
- Planning Organic Synthesis With "Reaction Maps"
- Alkene Addition Pattern #1: The "Carbocation Pathway"
- Alkene Addition Pattern #2: The "Three-Membered Ring" Pathway
- Alkene Addition Pattern #3: The "Concerted" Pathway
- Number Your Carbons!
- The 4 Major Classes of Reactions in Org 1
- How (and why) electrons flow
- Grossman's Rule
- Three Exam Tips
- A 3-Step Method For Thinking Through Synthesis Problems
- Putting It Together
- Putting Diels-Alder Products in Perspective
- The Ups and Downs of Cyclohexanes
- The Most Annoying Exceptions in Org 1 (Part 1)
- The Most Annoying Exceptions in Org 1 (Part 2)
- The Marriage May Be Bad, But the Divorce Still Costs Money
- 9 Nomenclature Conventions To Know
- Nucleophile attacks Electrophile
27 Case Studies of Successful O-Chem Students
- Success Stories: How Corina Got The The "Hard" Professor - And Got An A+ Anyway
- How Helena Aced Organic Chemistry
- From a "Drop" To B+ in Org 2 – How A Hard Working Student Turned It Around
- How Serge Aced Organic Chemistry
- Success Stories: How Zach Aced Organic Chemistry 1
- Success Stories: How Kari Went From C– to B+
- How Esther Bounced Back From a "C" To Get A's In Organic Chemistry 1 And 2
- How Tyrell Got The Highest Grade In Her Organic Chemistry Course
- This Is Why Students Use Flashcards
- Success Stories: How Stu Aced Organic Chemistry
- How John Pulled Up His Organic Chemistry Exam Grades
- Success Stories: How Nathan Aced Organic Chemistry (Without It Taking Over His Life)
- How Chris Aced Org 1 and Org 2
- Interview: How Jay Got an A+ In Organic Chemistry
- How to Do Well in Organic Chemistry: One Student's Advice
- "America's Top TA" Shares His Secrets For Teaching O-Chem
- "Organic Chemistry Is Like..." - A Few Metaphors
- How To Do Well In Organic Chemistry: Advice From A Tutor
- Guest post: "I went from being afraid of tests to actually looking forward to them".
Like Dipita Karmakar, I also do not understand why electrons in non-bonding orbitals are destabilising, making the compound anti-aromatic, rather than just non-aromatic. I would think that these electrons contribute to neither stabilisation or destabilisation.
I also do not understand how I would know from the Frost diagram, why, if I take a 4n+2 system, and remove 2 electrons, the result would be the anti-aromatic system expected from a 4n compound. Because all filled orbitals are stabilising? I’m not sure how to see the aromatic stabilisation or lack thereof in this context.
The key issue is not so much that they are in non-bonding orbitals, it is more that these non-bonding orbitals are singly-occupied (due to Hund’s rule).
This means that these compounds are very similar in behavior to free radicals – except because there are two free radicals, they are what are known as “diradicals”.
So imagine the instability of free radicals, times two.
(The barrier to recombination of free radicals is basically zero, so these types of compounds very readily combine with each other even if they manage to form – they also react rapidly with oxygen)
Just realised my mistake! It’s the number of pi electrons that tell you if it is aromatic or antiaromatic.
4n+2 is aromatic
4n is antiaromatic.
So to conclude: fulfilling all of Huckel’s Rules means a molecule is aromatic or antiaromatic. Drawing a Frost Circle can then determine whether a molecule is aromatic or whether it is antiaromatic.
Is this correct?
The Frost circle is mostly useful for visualizing what the energy levels will look like.
Once you know that the energy levels look like, then you fill them up with the number of available pi electrons.
When you do this with cyclobutadiene you end up with one doubly-occupied orbital and two singly-occupied orbitals (unstable). But when you do this with benzene you end up with three doubly-filled orbitals (stable!)
TYPO: Note 2 line 1 “The stability arising from antiaromaticity in the cyclobutadiene dication ” should refer to aromaticity.
Overall this is an excellent series of articles.
Fixed it, thank you!
Your diagram of the cyclooctatetraene dianion shows the added electrons in a non-bonding orbital. With 10 electrons it would obey the numerology of Huckel’s rule but the diagram argues against it with the added electrons in the non-bonding orbitals.
The compound has been made as a disodium salt but the most recent theory is that it exists because it is stabilized by interactions with counterions: J. Chem. Theory Comput. 2013, 9, 4436−4443.
“Like many multiply charged anions, COT2− exists in isolation only as a short-lived resonance state lying above neutral COT.”
“….Charge-compensating complexation of COT2−with two sodium cations results in a thermodynamically stable Na2COT compound”
Very interesting! Also from the paper: “Comparison with all-trans octatetraene indicates that COT2− has a substantial aromatic stabilization energy (25 kcal mol−1) approaching that of benzene (33 kcal mol−1), but this favorable influence is swamped by Coulomb repulsion. “
Is cyclobutadienyl anion aromatic or not
Cyclobutadienyl di-anion, yes, because it would have six pi electrons. Cyclobutadienyl anion, no, because it can only be formed from deprotonation of C-H and the negative charge (and ensuing lone pair) would be at right angles to the pi system.
How to make molecular orbital energy level of non-cyclic organic compounds?? Is there any perfect method for all compounds?? Please describe
If we’re strictly discussing pi systems, I suggest looking here: https://www.masterorganicchemistry.com/2017/02/16/molecular-orbitals-of-the-allyl-cation-allyl-radical-and-allyl-anion/